
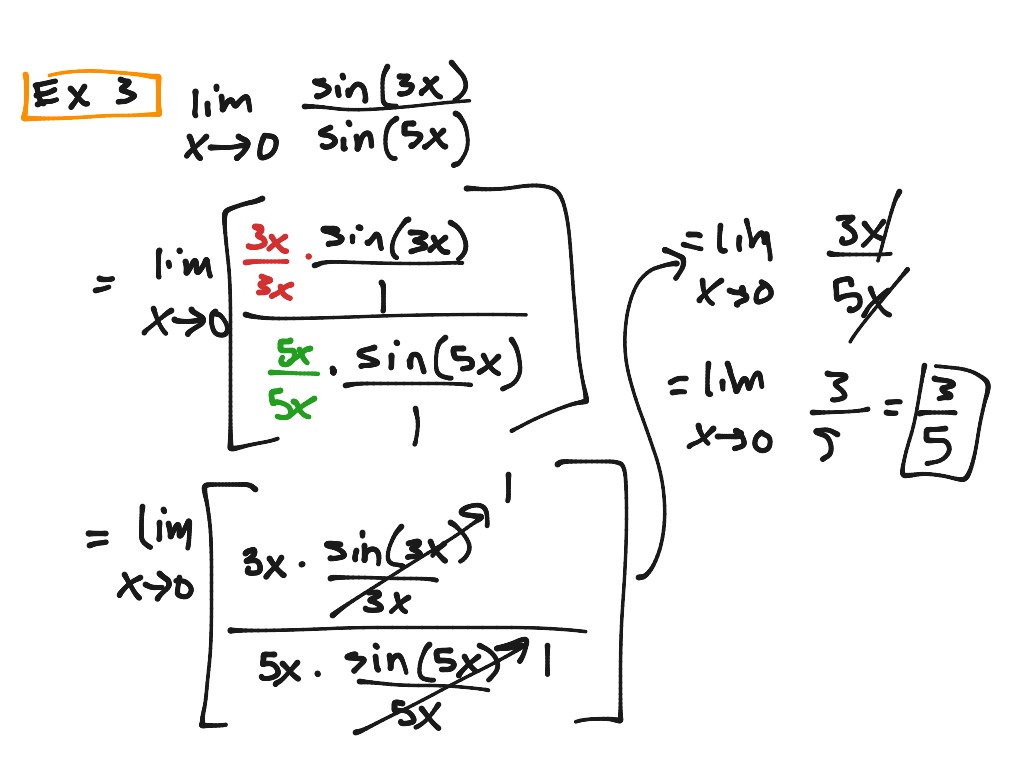
Now by computing the limit we have the form, therefore we can apply L’Hôpital’s rule and we get: Here we have the indeterminate form, to use L’Hôpital’s rule we re-write the expression as follow:

LIMITS IN CALCULUS HOW TO
Let’s see some examples of how to do that!!! In this case, after we get the derivatives of the quotient, we still get the indeterminate form of the type so we apply L’Hôpital’s Rule again, and therefore we get:įor other Indeterminate forms, we have to do some transformation on the expression to bring it to one of the two forms that L’Hôpital’s rule solves. Then we calculate the limit of the derivatives of the quotient of and, i.e., Where is a real number or infinity, and if we have one of the following cases: L’Hôpital’s rule is a method used to evaluate limits when we have the case of a quotient of two functions giving us the indeterminate form of the type or. L’Hôpital’s rule and how to solve indeterminate forms Here are some examples to illustrate each of these indeterminate cases: In total there is seven indeterminate forms, here they are: We call an indeterminate form, when computing limits the case when we get an expression that we cannot determine the limit. This occurs with trigonometric functions.While studying calculus or other branches of mathematics we may need to find the limits of a function, a sequence, or an expression, and in doing so we stumble on a situation where we cannot determine the limits, in this article we will learn about the different indeterminate forms and how to work around them in order to find the limits we are looking for.
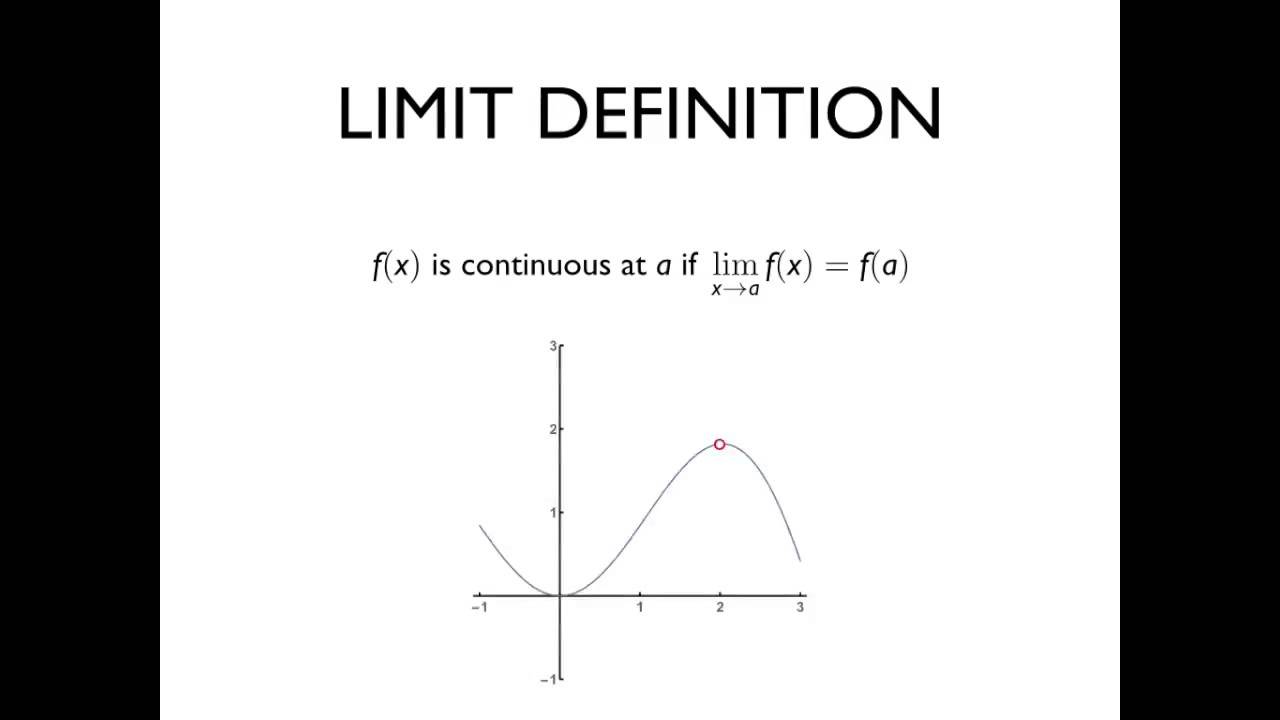
Finally, the last reason for a limit to not exist would be for the function to wiggle from one point to the next, never reaching a single numeric value. This occurs when a function has a vertical asymptote at x=a causing the function to increase or decrease indefinitely. Another reason would occur if a function increases or decreases indefinitely at a given x-value (Stewart 61). This causes the left and right hand limits to not be equal therefore causing the limit to not exist. Another reason for a limit wouldn"t exist would be for a graph to break, if the pieces don"t meet up at an intended height. Since, we already know about the rule of left and right hand limits so we"re one third of the way there. So now that we know when a Limit exists, we need to know when a limit doesn"t exist. Lim f(x) = L = Lim f(x) = L then Lim f(x) = L. This concept is called Left and Right Hand Limits. First, the Limit of a point only exists when the limits from the approaching the point from the left(-) equals the limit of the point from the right(+). Now Limits have certain rules to follow if they want to be Limits. Which in English means, we can make f(x) as close to L as we can by taking x sufficiently close to a, but not equal to a (Stewart 91). By using f(x), a, and L we say that, "the Limit of f(x), as x approaches a, equals L" (Stewart 91). Limits are very important in all fields involving any form of math, whether solving the acceleration of a rocket ship to analyzing and interpolation stock market data.Ī Limit is the height a function Intends to reach at a given x value, whether or not it actually reaches it (Kelly 57). The purpose of this essay is to discuss and teach the concepts of Limits and why they exist. During many Calculus courses the Concept of Limits are taught to students by showing them how, but not why.


 0 kommentar(er)
0 kommentar(er)
